unit 5 polynomial functions homework 2 graphing polynomial functions answers

• BLIZZARD BAG
• Table of Contents
• 1.1 Multiple Representations
• 1.2 Linear Functions and Regression
• ​1.3 Factoring
• Unit 1 Review
• 2.1 Function Introduction
• 2.2 Domain & Range Graphically
• 2.3 Limits Graphically
• 2.4 Limits to Infinity
• Unit 2 Review
• 3.1 Domains & DIscontinuities
• 3.2 Extrema
• 3.3 Piecewise Functions
• Unit 3 Review
• 4.1 Transformations
• 4.2 Even And Odd Functions
• 4.3 Operations with Funcitons
• 4.4 Inverse Functions
• Unit 4 Review
• 5.1 Operations of Polynomials
• 5.2 Polynomial Division & Factor Theroem
• 5.3 Polynomial Graphs
• Unit 5 Review
• 6.1 Solving Rational Equations
• 6.2 Complex Fractions & Rationalizing
• 6.3 Graphing Rational Functions
• 6.4 Variation & Modeling
• Unit 6 Review
• 7.1 Exponential Functions
• 7.2 Logarithmic Functions
• 7.3 Exp & Log Problem Solving
• Extra Practice
• What's the deal with e?
• Unit 7 Review
• Matrix Operations
• Matrix Multiplication
• Inverse Matrices
• Matrices Review
• Midterm Review
• 8.1 Angles and Degrees
• 8.2 Angles & Radians
• 8.3 Velocity
• 8.4 Basic Trig Functions
• Unit 8 Review
• 9.1 Reference Triangles
• Unit Circle Table
• 9.3 Evaluating Trig Functions
• Unit 9 Review
• 10.1 - Graphing Sine & Cosine
• 10.2 - Phase Shift & Tangent
• ​10.3 - Reciprocal Trig Graphs
• 10.4 - Inverse Trig Functions
• 10.1 - Parents of Sine & Cosine
• 10.2 - Graphing Sine & Cosine
• 10.3 - Parents of Tangent & Cotangent
• 10.4 - Graphing Tangent & Cotangent
• 10.5 - Parents of Secant & Cosecant
• 10.6 - Graphing Secant & Cosecant
• 10.7 - Inverse Trig Functions
• Unit 10 Review
• Cumulative Review
• Sample Terms Quiz
• 11.1 Basic Trig Ids
• 11.2 - Verifying Trig IDs
• 11.3 - Sum & Difference Trig IDs
• 11.4 - Double & Half Angle Trig IDs
• Unit 11 Review
• 12.1 - Law of Sines
• 12.2 - Law of Cosines
• 12.3 - Area of Triangles
• Review Unit 12
• 13.1 - 3D Graphs
• 13.2 - Polar Coordinates
• Unit 13 Review
• 14.1 - Sequences, Series & Summation
• 14.2 - Arithmetic & Geometric Sequences/Series
• 14.3 - Binomial Theorem
• Unit 14 Review
• Final Review
• 5.2 Polynomial Division & Factor Theorem
• Test Unit 5

5.3 Graphs of Polynomial Functions

Learning objectives.

In this section, you will:

• Recognize characteristics of graphs of polynomial functions.
• Use factoring to find zeros of polynomial functions.
• Identify zeros and their multiplicities.
• Determine end behavior.
• Understand the relationship between degree and turning points.
• Graph polynomial functions.
• Use the Intermediate Value Theorem.

The revenue in millions of dollars for a fictional cable company from 2006 through 2013 is shown in Table 1 .

The revenue can be modeled by the polynomial function

where R R represents the revenue in millions of dollars and t t represents the year, with t = 6 t = 6 corresponding to 2006. Over which intervals is the revenue for the company increasing? Over which intervals is the revenue for the company decreasing? These questions, along with many others, can be answered by examining the graph of the polynomial function. We have already explored the local behavior of quadratics, a special case of polynomials. In this section we will explore the local behavior of polynomials in general.

Recognizing Characteristics of Graphs of Polynomial Functions

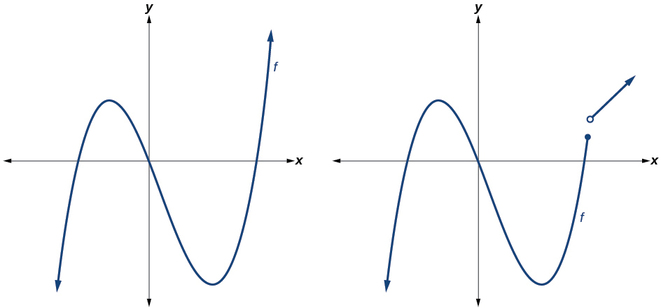
Polynomial functions of degree 2 or more have graphs that do not have sharp corners; recall that these types of graphs are called smooth curves. Polynomial functions also display graphs that have no breaks. Curves with no breaks are called continuous. Figure 1 shows a graph that represents a polynomial function and a graph that represents a function that is not a polynomial.

Recognizing Polynomial Functions

Which of the graphs in Figure 2 represents a polynomial function?

The graphs of f f and h h are graphs of polynomial functions. They are smooth and continuous .

The graphs of g g and k k are graphs of functions that are not polynomials. The graph of function g g has a sharp corner. The graph of function k k is not continuous.

Do all polynomial functions have as their domain all real numbers?

Yes. Any real number is a valid input for a polynomial function.

Using Factoring to Find Zeros of Polynomial Functions

Recall that if f f is a polynomial function, the values of x x for which f ( x ) = 0 f ( x ) = 0 are called zeros of f . f . If the equation of the polynomial function can be factored, we can set each factor equal to zero and solve for the zeros .

We can use this method to find x - x - intercepts because at the x - x - intercepts we find the input values when the output value is zero. For general polynomials, this can be a challenging prospect. While quadratics can be solved using the relatively simple quadratic formula, the corresponding formulas for cubic and fourth-degree polynomials are not simple enough to remember, and formulas do not exist for general higher-degree polynomials. Consequently, we will limit ourselves to three cases:

• The polynomial can be factored using known methods: greatest common factor and trinomial factoring.
• The polynomial is given in factored form.
• Technology is used to determine the intercepts.

Given a polynomial function f , f , find the x -intercepts by factoring.

• Set f ( x ) = 0. f ( x ) = 0.
• Factor out any common monomial factors.
• Factor any factorable binomials or trinomials.
• Set each factor equal to zero and solve to find the x - x - intercepts.

Finding the x -Intercepts of a Polynomial Function by Factoring

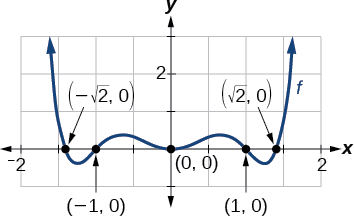
Find the x -intercepts of f ( x ) = x 6 − 3 x 4 + 2 x 2 . f ( x ) = x 6 − 3 x 4 + 2 x 2 .

We can attempt to factor this polynomial to find solutions for f ( x ) = 0. f ( x ) = 0.

This gives us five x -intercepts: ( 0 , 0 ) , ( 1 , 0 ) , ( −1 , 0 ) , ( 2 , 0 ) , ( 0 , 0 ) , ( 1 , 0 ) , ( −1 , 0 ) , ( 2 , 0 ) , and ( − 2 , 0 ) . ( − 2 , 0 ) . See Figure 3 . We can see that this is an even function because it is symmetric about the y -axis.

Find the x -intercepts of f ( x ) = x 3 − 5 x 2 − x + 5. f ( x ) = x 3 − 5 x 2 − x + 5.

Find solutions for f ( x ) = 0 f ( x ) = 0 by factoring.

There are three x -intercepts: ( −1 , 0 ) , ( 1 , 0 ) , ( −1 , 0 ) , ( 1 , 0 ) , and ( 5 , 0 ) . ( 5 , 0 ) . See Figure 4 .

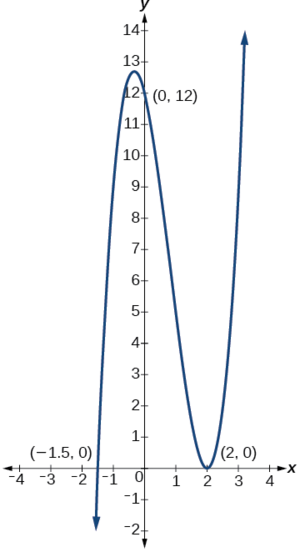
Finding the y - and x -Intercepts of a Polynomial in Factored Form

Find the y - and x -intercepts of g ( x ) = ( x − 2 ) 2 ( 2 x + 3 ) . g ( x ) = ( x − 2 ) 2 ( 2 x + 3 ) .

The y -intercept can be found by evaluating g ( 0 ) . g ( 0 ) .

So the y -intercept is ( 0 , 12 ) . ( 0 , 12 ) .

The x -intercepts can be found by solving g ( x ) = 0. g ( x ) = 0.

So the x -intercepts are ( 2 , 0 ) ( 2 , 0 ) and ( − 3 2 , 0 ) . ( − 3 2 , 0 ) .

We can always check that our answers are reasonable by using a graphing calculator to graph the polynomial as shown in Figure 5 .

Finding the x -Intercepts of a Polynomial Function Using a Graph

Find the x -intercepts of h ( x ) = x 3 + 4 x 2 + x − 6. h ( x ) = x 3 + 4 x 2 + x − 6.

This polynomial is not in factored form, has no common factors, and does not appear to be factorable using techniques previously discussed. Fortunately, we can use technology to find the intercepts. Keep in mind that some values make graphing difficult by hand. In these cases, we can take advantage of graphing utilities.

Looking at the graph of this function, as shown in Figure 6 , it appears that there are x -intercepts at x = −3 , −2 , x = −3 , −2 , and 1. 1.

We can check whether these are correct by substituting these values for x x and verifying that

Since h ( x ) = x 3 + 4 x 2 + x − 6 , h ( x ) = x 3 + 4 x 2 + x − 6 , we have:

Each x -intercept corresponds to a zero of the polynomial function and each zero yields a factor, so we can now write the polynomial in factored form.

Find the y - and x -intercepts of the function f ( x ) = x 4 − 19 x 2 + 30 x . f ( x ) = x 4 − 19 x 2 + 30 x .

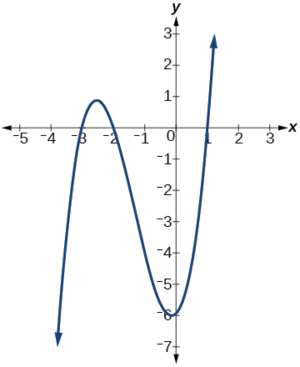
Identifying Zeros and Their Multiplicities

Graphs behave differently at various x -intercepts. Sometimes, the graph will cross over the horizontal axis at an intercept. Other times, the graph will touch the horizontal axis and "bounce" off.

Suppose, for example, we graph the function shown.

Notice in Figure 7 that the behavior of the function at each of the x -intercepts is different.

The x -intercept x = −3 x = −3 is the solution of equation ( x + 3 ) = 0. ( x + 3 ) = 0. The graph passes directly through the x -intercept at x = −3. x = −3. The factor is linear (has a degree of 1), so the behavior near the intercept is like that of a line—it passes directly through the intercept. We call this a single zero because the zero corresponds to a single factor of the function.

The x -intercept x = 2 x = 2 is the repeated solution of equation ( x − 2 ) 2 = 0. ( x − 2 ) 2 = 0. The graph touches the axis at the intercept and changes direction. The factor is quadratic (degree 2), so the behavior near the intercept is like that of a quadratic—it bounces off of the horizontal axis at the intercept.

The factor is repeated, that is, the factor ( x − 2 ) ( x − 2 ) appears twice. The number of times a given factor appears in the factored form of the equation of a polynomial is called the multiplicity . The zero associated with this factor, x = 2 , x = 2 , has multiplicity 2 because the factor ( x − 2 ) ( x − 2 ) occurs twice.

The x -intercept x = − 1 x = − 1 is the repeated solution of factor ( x + 1 ) 3 = 0. ( x + 1 ) 3 = 0. The graph passes through the axis at the intercept, but flattens out a bit first. This factor is cubic (degree 3), so the behavior near the intercept is like that of a cubic—with the same S-shape near the intercept as the toolkit function f ( x ) = x 3 . f ( x ) = x 3 . We call this a triple zero, or a zero with multiplicity 3.

For zeros with even multiplicities, the graphs touch or are tangent to the x -axis. For zeros with odd multiplicities, the graphs cross or intersect the x -axis. See Figure 8 for examples of graphs of polynomial functions with multiplicity 1, 2, and 3.

For higher even powers, such as 4, 6, and 8, the graph will still touch and bounce off of the horizontal axis but, for each increasing even power, the graph will appear flatter as it approaches and leaves the x -axis.

For higher odd powers, such as 5, 7, and 9, the graph will still cross through the horizontal axis, but for each increasing odd power, the graph will appear flatter as it approaches and leaves the x -axis.

Graphical Behavior of Polynomials at x -Intercepts

If a polynomial contains a factor of the form ( x − h ) p , ( x − h ) p , the behavior near the x - x - intercept h h is determined by the power p . p . We say that x = h x = h is a zero of multiplicity p . p .

The graph of a polynomial function will touch the x -axis at zeros with even multiplicities. The graph will cross the x -axis at zeros with odd multiplicities.

The sum of the multiplicities is the degree of the polynomial function.

Given a graph of a polynomial function of degree n , n , identify the zeros and their multiplicities.

• If the graph crosses the x -axis and appears almost linear at the intercept, it is a single zero.
• If the graph touches the x -axis and bounces off of the axis, it is a zero with even multiplicity.
• If the graph crosses the x -axis at a zero, it is a zero with odd multiplicity.
• The sum of the multiplicities is ≤ n . ≤ n .

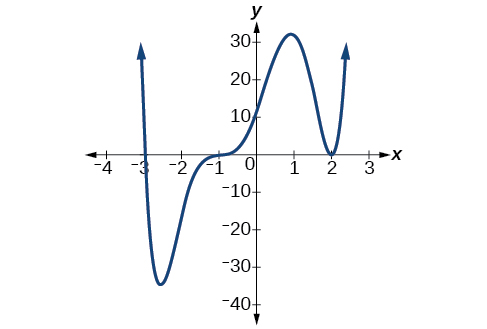
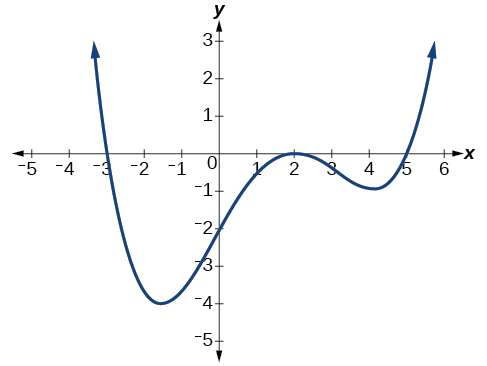
Use the graph of the function of degree 6 in Figure 9 to identify the zeros of the function and their possible multiplicities.

The polynomial function is of degree 6. The sum of the multiplicities must be 6.

Starting from the left, the first zero occurs at x = −3. x = −3. The graph touches the x -axis, so the multiplicity of the zero must be even. The zero of −3 −3 most likely has multiplicity 2. 2.

The next zero occurs at x = −1. x = −1. The graph looks almost linear at this point. This is a single zero of multiplicity 1.

The last zero occurs at x = 4. x = 4. The graph crosses the x -axis, so the multiplicity of the zero must be odd. We know that the multiplicity is likely 3 and that the sum of the multiplicities is 6.

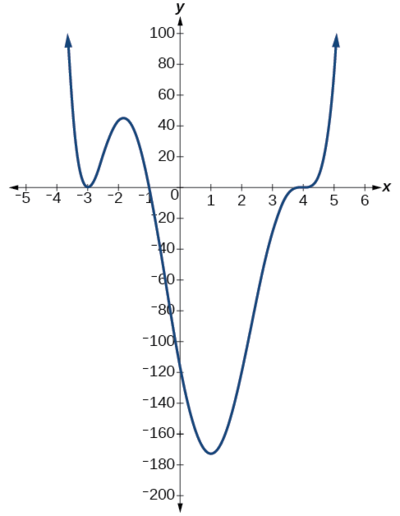
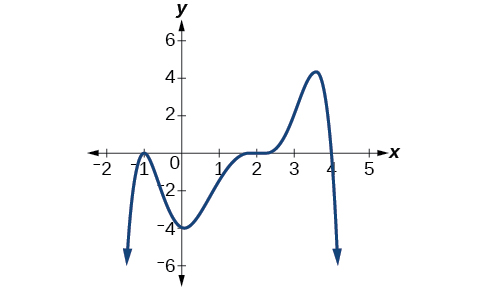
Use the graph of the function of degree 9 in Figure 10 to identify the zeros of the function and their multiplicities.

Determining End Behavior

As we have already learned, the behavior of a graph of a polynomial function of the form

will either ultimately rise or fall as x x increases without bound and will either rise or fall as x x decreases without bound. This is because for very large inputs, say 100 or 1,000, the leading term dominates the size of the output. The same is true for very small inputs, say –100 or –1,000.

Recall that we call this behavior the end behavior of a function. As we pointed out when discussing quadratic equations, when the leading term of a polynomial function, a n x n , a n x n , is an even power function, as x x increases or decreases without bound, f ( x ) f ( x ) increases without bound. When the leading term is an odd power function, as x x decreases without bound, f ( x ) f ( x ) also decreases without bound; as x x increases without bound, f ( x ) f ( x ) also increases without bound. If the leading term is negative, it will change the direction of the end behavior. Figure 11 summarizes all four cases.

Understanding the Relationship between Degree and Turning Points

In addition to the end behavior, recall that we can analyze a polynomial function’s local behavior. It may have a turning point where the graph changes from increasing to decreasing (rising to falling) or decreasing to increasing (falling to rising). Look at the graph of the polynomial function f ( x ) = x 4 − x 3 − 4 x 2 + 4 x f ( x ) = x 4 − x 3 − 4 x 2 + 4 x in Figure 12 . The graph has three turning points.

This function f f is a 4 th degree polynomial function and has 3 turning points. The maximum number of turning points of a polynomial function is always one less than the degree of the function.

Interpreting Turning Points

A turning point is a point of the graph where the graph changes from increasing to decreasing (rising to falling) or decreasing to increasing (falling to rising).

A polynomial of degree n n will have at most n − 1 n − 1 turning points.

Finding the Maximum Number of Turning Points Using the Degree of a Polynomial Function

Find the maximum number of turning points of each polynomial function.

• ⓐ f ( x ) = − x 3 + 4 x 5 − 3 x 2 + 1 f ( x ) = − x 3 + 4 x 5 − 3 x 2 + 1
• ⓑ f ( x ) = − ( x − 1 ) 2 ( 1 + 2 x 2 ) f ( x ) = − ( x − 1 ) 2 ( 1 + 2 x 2 )

First, rewrite the polynomial function in descending order: f ( x ) = 4 x 5 − x 3 − 3 x 2 + 1 f ( x ) = 4 x 5 − x 3 − 3 x 2 + 1

Identify the degree of the polynomial function. This polynomial function is of degree 5.

The maximum number of turning points is 5 − 1 = 4. 5 − 1 = 4.

First, identify the leading term of the polynomial function if the function were expanded.

Then, identify the degree of the polynomial function. This polynomial function is of degree 4.

The maximum number of turning points is 4 − 1 = 3. 4 − 1 = 3.

Graphing Polynomial Functions

We can use what we have learned about multiplicities, end behavior, and turning points to sketch graphs of polynomial functions. Let us put this all together and look at the steps required to graph polynomial functions.

Given a polynomial function, sketch the graph.

• Find the intercepts.
• Check for symmetry. If the function is an even function, its graph is symmetrical about the y - y - axis, that is, f ( − x ) = f ( x ) . f ( − x ) = f ( x ) . If a function is an odd function, its graph is symmetrical about the origin, that is, f ( − x ) = − f ( x ) . f ( − x ) = − f ( x ) .
• Use the multiplicities of the zeros to determine the behavior of the polynomial at the x - x - intercepts.
• Determine the end behavior by examining the leading term.
• Use the end behavior and the behavior at the intercepts to sketch a graph.
• Ensure that the number of turning points does not exceed one less than the degree of the polynomial.
• Optionally, use technology to check the graph.

Sketching the Graph of a Polynomial Function

Sketch a graph of f ( x ) = −2 ( x + 3 ) 2 ( x − 5 ) . f ( x ) = −2 ( x + 3 ) 2 ( x − 5 ) .

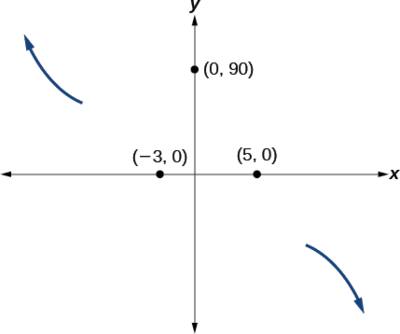
This graph has two x -intercepts. At x = −3 , x = −3 , the factor is squared, indicating a multiplicity of 2. The graph will bounce at this x -intercept. At x = 5 , x = 5 , the function has a multiplicity of one, indicating the graph will cross through the axis at this intercept.

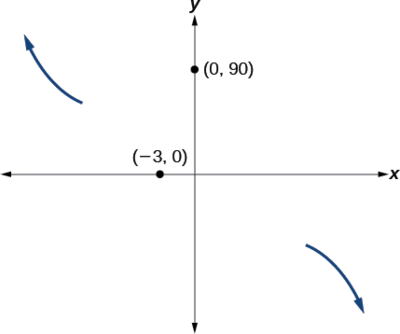
The y -intercept is found by evaluating f ( 0 ) . f ( 0 ) .

The y -intercept is ( 0 , 90 ) . ( 0 , 90 ) .

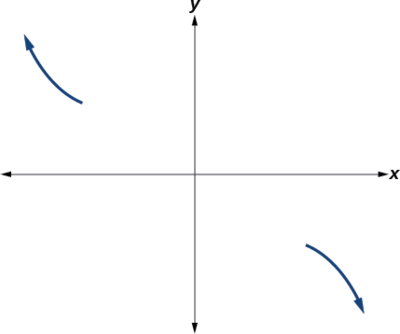
Additionally, we can see the leading term, if this polynomial were multiplied out, would be − 2 x 3 , − 2 x 3 , so the end behavior is that of a vertically reflected cubic, with the outputs decreasing as the inputs approach infinity, and the outputs increasing as the inputs approach negative infinity. See Figure 13 .

To sketch this, we consider that:

• As x → − ∞ x → − ∞ the function f ( x ) → ∞ , f ( x ) → ∞ , so we know the graph starts in the second quadrant and is decreasing toward the x - x - axis.
• Since f ( − x ) = −2 ( − x + 3 ) 2 ( − x – 5 ) f ( − x ) = −2 ( − x + 3 ) 2 ( − x – 5 ) is not equal to f ( x ) , f ( x ) , the graph does not display symmetry.

At ( 0 , 90 ) , ( 0 , 90 ) , the graph crosses the y -axis at the y -intercept. See Figure 14 .

Somewhere after this point, the graph must turn back down or start decreasing toward the horizontal axis because the graph passes through the next intercept at ( 5 , 0 ) . ( 5 , 0 ) . See Figure 15 .

As x → ∞ x → ∞ the function f ( x ) → −∞ , f ( x ) → −∞ , so we know the graph continues to decrease, and we can stop drawing the graph in the fourth quadrant.

Using technology, we can create the graph for the polynomial function, shown in Figure 16 , and verify that the resulting graph looks like our sketch in Figure 15 .

Sketch a graph of f ( x ) = 1 4 x ( x − 1 ) 4 ( x + 3 ) 3 . f ( x ) = 1 4 x ( x − 1 ) 4 ( x + 3 ) 3 .

Using the Intermediate Value Theorem

In some situations, we may know two points on a graph but not the zeros. If those two points are on opposite sides of the x -axis, we can confirm that there is a zero between them. Consider a polynomial function f f whose graph is smooth and continuous. The Intermediate Value Theorem states that for two numbers a a and b b in the domain of f , f , if a < b a < b and f ( a ) ≠ f ( b ) , f ( a ) ≠ f ( b ) , then the function f f takes on every value between f ( a ) f ( a ) and f ( b ) . f ( b ) . (While the theorem is intuitive, the proof is actually quite complicated and requires higher mathematics.) We can apply this theorem to a special case that is useful in graphing polynomial functions. If a point on the graph of a continuous function f f at x = a x = a lies above the x - x - axis and another point at x = b x = b lies below the x - x - axis, there must exist a third point between x = a x = a and x = b x = b where the graph crosses the x - x - axis. Call this point ( c , f ( c ) ) . ( c , f ( c ) ) . This means that we are assured there is a solution c c where f ( c ) = 0. f ( c ) = 0.

In other words, the Intermediate Value Theorem tells us that when a polynomial function changes from a negative value to a positive value, the function must cross the x - x - axis. Figure 17 shows that there is a zero between a a and b . b .

• Intermediate Value Theorem

Let f f be a polynomial function. The Intermediate Value Theorem states that if f ( a ) f ( a ) and f ( b ) f ( b ) have opposite signs, then there exists at least one value c c between a a and b b for which f ( c ) = 0. f ( c ) = 0.

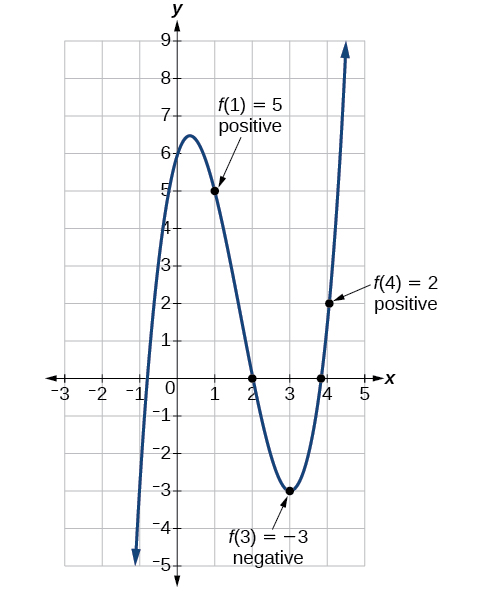
Show that the function f ( x ) = x 3 − 5 x 2 + 3 x + 6 f ( x ) = x 3 − 5 x 2 + 3 x + 6 has at least two real zeros between x = 1 x = 1 and x = 4. x = 4.

As a start, evaluate f ( x ) f ( x ) at the integer values x = 1 , 2 , 3 , x = 1 , 2 , 3 , and 4. 4. See Table 2 .

We see that one zero occurs at x = 2. x = 2. Also, since f ( 3 ) f ( 3 ) is negative and f ( 4 ) f ( 4 ) is positive, by the Intermediate Value Theorem, there must be at least one real zero between 3 and 4.

We have shown that there are at least two real zeros between x = 1 x = 1 and x = 4. x = 4.

We can also see on the graph of the function in Figure 18 that there are two real zeros between x = 1 x = 1 and x = 4. x = 4.

Show that the function f ( x ) = 7 x 5 − 9 x 4 − x 2 f ( x ) = 7 x 5 − 9 x 4 − x 2 has at least one real zero between x = 1 x = 1 and x = 2. x = 2.

Writing Formulas for Polynomial Functions

Now that we know how to find zeros of polynomial functions, we can use them to write formulas based on graphs. Because a polynomial function written in factored form will have an x -intercept where each factor is equal to zero, we can form a function that will pass through a set of x -intercepts by introducing a corresponding set of factors.

Factored Form of Polynomials

If a polynomial of lowest degree p p has horizontal intercepts at x = x 1 , x 2 , … , x n , x = x 1 , x 2 , … , x n , then the polynomial can be written in the factored form: f ( x ) = a ( x − x 1 ) p 1 ( x − x 2 ) p 2 ⋯ ( x − x n ) p n f ( x ) = a ( x − x 1 ) p 1 ( x − x 2 ) p 2 ⋯ ( x − x n ) p n where the powers p i p i on each factor can be determined by the behavior of the graph at the corresponding intercept, and the stretch factor a a can be determined given a value of the function other than the x -intercept.

Given a graph of a polynomial function, write a formula for the function.

• Identify the x -intercepts of the graph to find the factors of the polynomial.
• Examine the behavior of the graph at the x -intercepts to determine the multiplicity of each factor.
• Find the polynomial of least degree containing all the factors found in the previous step.
• Use any other point on the graph (the y -intercept may be easiest) to determine the stretch factor.

Writing a Formula for a Polynomial Function from the Graph

Write a formula for the polynomial function shown in Figure 19 .

This graph has three x -intercepts: x = −3 , 2 , x = −3 , 2 , and 5. 5. The y -intercept is located at ( 0 , 2 ) . ( 0 , 2 ) . At x = −3 x = −3 and x = 5 , x = 5 , the graph passes through the axis linearly, suggesting the corresponding factors of the polynomial will be linear. At x = 2 , x = 2 , the graph bounces at the intercept, suggesting the corresponding factor of the polynomial will be second degree (quadratic). Together, this gives us

To determine the stretch factor, we utilize another point on the graph. We will use the y - y - intercept ( 0 , – 2 ) , ( 0 , – 2 ) , to solve for a . a .

The graphed polynomial appears to represent the function f ( x ) = 1 30 ( x + 3 ) ( x − 2 ) 2 ( x − 5 ) . f ( x ) = 1 30 ( x + 3 ) ( x − 2 ) 2 ( x − 5 ) .

Given the graph shown in Figure 20 , write a formula for the function shown.

Using Local and Global Extrema

With quadratics, we were able to algebraically find the maximum or minimum value of the function by finding the vertex. For general polynomials, finding these turning points is not possible without more advanced techniques from calculus. Even then, finding where extrema occur can still be algebraically challenging. For now, we will estimate the locations of turning points using technology to generate a graph.

Each turning point represents a local minimum or maximum. Sometimes, a turning point is the highest or lowest point on the entire graph. In these cases, we say that the turning point is a global maximum or a global minimum . These are also referred to as the absolute maximum and absolute minimum values of the function.

Local and Global Extrema

A local maximum or local minimum at x = a x = a (sometimes called the relative maximum or minimum, respectively) is the output at the highest or lowest point on the graph in an open interval around x = a . x = a . If a function has a local maximum at a , a , then f ( a ) ≥ f ( x ) f ( a ) ≥ f ( x ) for all x x in an open interval around x = a . x = a . If a function has a local minimum at a , a , then f ( a ) ≤ f ( x ) f ( a ) ≤ f ( x ) for all x x in an open interval around x = a . x = a .

A global maximum or global minimum is the output at the highest or lowest point of the function. If a function has a global maximum at a , a , then f ( a ) ≥ f ( x ) f ( a ) ≥ f ( x ) for all x . x . If a function has a global minimum at a , a , then f ( a ) ≤ f ( x ) f ( a ) ≤ f ( x ) for all x . x .

We can see the difference between local and global extrema in Figure 21 .

Do all polynomial functions have a global minimum or maximum?

No. Only polynomial functions of even degree have a global minimum or maximum. For example, f ( x ) = x f ( x ) = x has neither a global maximum nor a global minimum.

Using Local Extrema to Solve Applications

An open-top box is to be constructed by cutting out squares from each corner of a 14 cm by 20 cm sheet of plastic and then folding up the sides. Find the size of squares that should be cut out to maximize the volume enclosed by the box.

We will start this problem by drawing a picture like that in Figure 22 , labeling the width of the cut-out squares with a variable, w . w .

Notice that after a square is cut out from each end, it leaves a ( 14 − 2 w ) ( 14 − 2 w ) cm by ( 20 − 2 w ) ( 20 − 2 w ) cm rectangle for the base of the box, and the box will be w w cm tall. This gives the volume

Notice, since the factors are w , w , 20 – 2 w 20 – 2 w and 14 – 2 w , 14 – 2 w , the three zeros are 10, 7, and 0, respectively. Because a height of 0 cm is not reasonable, we consider the only the zeros 10 and 7. The shortest side is 14 and we are cutting off two squares, so values w w may take on are greater than zero or less than 7. This means we will restrict the domain of this function to 0 < w < 7. 0 < w < 7. Using technology to sketch the graph of V ( w ) V ( w ) on this reasonable domain, we get a graph like that in Figure 23 . We can use this graph to estimate the maximum value for the volume, restricted to values for w w that are reasonable for this problem—values from 0 to 7.

From this graph, we turn our focus to only the portion on the reasonable domain, [ 0 , 7 ] . [ 0 , 7 ] . We can estimate the maximum value to be around 340 cubic cm, which occurs when the squares are about 2.75 cm on each side. To improve this estimate, we could use advanced features of our technology, if available, or simply change our window to zoom in on our graph to produce Figure 24 .

From this zoomed-in view, we can refine our estimate for the maximum volume to about 339 cubic cm, when the squares measure approximately 2.7 cm on each side.

Use technology to find the maximum and minimum values on the interval [ −1 , 4 ] [ −1 , 4 ] of the function f ( x ) = − 0.2 ( x − 2 ) 3 ( x + 1 ) 2 ( x − 4 ) . f ( x ) = − 0.2 ( x − 2 ) 3 ( x + 1 ) 2 ( x − 4 ) .

Access the following online resource for additional instruction and practice with graphing polynomial functions.

5.3 Section Exercises

What is the difference between an x - x - intercept and a zero of a polynomial function f ? f ?

If a polynomial function of degree n n has n n distinct zeros, what do you know about the graph of the function?

Explain how the Intermediate Value Theorem can assist us in finding a zero of a function.

Explain how the factored form of the polynomial helps us in graphing it.

If the graph of a polynomial just touches the x -axis and then changes direction, what can we conclude about the factored form of the polynomial?

For the following exercises, find the x - x - or t -intercepts of the polynomial functions.

C ( t ) = 2 ( t − 4 ) ( t + 1 ) ( t − 6 ) C ( t ) = 2 ( t − 4 ) ( t + 1 ) ( t − 6 )

C ( t ) = 3 ( t + 2 ) ( t − 3 ) ( t + 5 ) C ( t ) = 3 ( t + 2 ) ( t − 3 ) ( t + 5 )

C ( t ) = 4 t ( t − 2 ) 2 ( t + 1 ) C ( t ) = 4 t ( t − 2 ) 2 ( t + 1 )

C ( t ) = 2 t ( t − 3 ) ( t + 1 ) 2 C ( t ) = 2 t ( t − 3 ) ( t + 1 ) 2

C ( t ) = 2 t 4 − 8 t 3 + 6 t 2 C ( t ) = 2 t 4 − 8 t 3 + 6 t 2

C ( t ) = 4 t 4 + 12 t 3 − 40 t 2 C ( t ) = 4 t 4 + 12 t 3 − 40 t 2

f ( x ) = x 4 − x 2 f ( x ) = x 4 − x 2

f ( x ) = x 3 + x 2 − 20 x f ( x ) = x 3 + x 2 − 20 x

f ( x ) = x 3 + 6 x 2 − 7 x f ( x ) = x 3 + 6 x 2 − 7 x

f ( x ) = x 3 + x 2 − 4 x − 4 f ( x ) = x 3 + x 2 − 4 x − 4

f ( x ) = x 3 + 2 x 2 − 9 x − 18 f ( x ) = x 3 + 2 x 2 − 9 x − 18

f ( x ) = 2 x 3 − x 2 − 8 x + 4 f ( x ) = 2 x 3 − x 2 − 8 x + 4

f ( x ) = x 6 − 7 x 3 − 8 f ( x ) = x 6 − 7 x 3 − 8

f ( x ) = 2 x 4 + 6 x 2 − 8 f ( x ) = 2 x 4 + 6 x 2 − 8

f ( x ) = x 3 − 3 x 2 − x + 3 f ( x ) = x 3 − 3 x 2 − x + 3

f ( x ) = x 6 − 2 x 4 − 3 x 2 f ( x ) = x 6 − 2 x 4 − 3 x 2

f ( x ) = x 6 − 3 x 4 − 4 x 2 f ( x ) = x 6 − 3 x 4 − 4 x 2

f ( x ) = x 5 − 5 x 3 + 4 x f ( x ) = x 5 − 5 x 3 + 4 x

For the following exercises, use the Intermediate Value Theorem to confirm that the given polynomial has at least one zero within the given interval.

f ( x ) = x 3 − 9 x , f ( x ) = x 3 − 9 x , between x = −4 x = −4 and x = −2. x = −2.

f ( x ) = x 3 − 9 x , f ( x ) = x 3 − 9 x , between x = 2 x = 2 and x = 4. x = 4.

f ( x ) = x 5 − 2 x , f ( x ) = x 5 − 2 x , between x = 1 x = 1 and x = 2. x = 2.

f ( x ) = − x 4 + 4 , f ( x ) = − x 4 + 4 , between x = 1 x = 1 and x = 3 x = 3 .

f ( x ) = −2 x 3 − x , f ( x ) = −2 x 3 − x , between x = –1 x = –1 and x = 1. x = 1.

f ( x ) = x 3 − 100 x + 2 , f ( x ) = x 3 − 100 x + 2 , between x = 0.01 x = 0.01 and x = 0.1 x = 0.1

For the following exercises, find the zeros and give the multiplicity of each.

f ( x ) = ( x + 2 ) 3 ( x − 3 ) 2 f ( x ) = ( x + 2 ) 3 ( x − 3 ) 2

f ( x ) = x 2 ( 2 x + 3 ) 5 ( x − 4 ) 2 f ( x ) = x 2 ( 2 x + 3 ) 5 ( x − 4 ) 2

f ( x ) = x 3 ( x − 1 ) 3 ( x + 2 ) f ( x ) = x 3 ( x − 1 ) 3 ( x + 2 )

f ( x ) = x 2 ( x 2 + 4 x + 4 ) f ( x ) = x 2 ( x 2 + 4 x + 4 )

f ( x ) = ( 2 x + 1 ) 3 ( 9 x 2 − 6 x + 1 ) f ( x ) = ( 2 x + 1 ) 3 ( 9 x 2 − 6 x + 1 )

f ( x ) = ( 3 x + 2 ) 5 ( x 2 − 10 x + 25 ) f ( x ) = ( 3 x + 2 ) 5 ( x 2 − 10 x + 25 )

f ( x ) = x ( 4 x 2 − 12 x + 9 ) ( x 2 + 8 x + 16 ) f ( x ) = x ( 4 x 2 − 12 x + 9 ) ( x 2 + 8 x + 16 )

f ( x ) = x 6 − x 5 − 2 x 4 f ( x ) = x 6 − x 5 − 2 x 4

f ( x ) = 3 x 4 + 6 x 3 + 3 x 2 f ( x ) = 3 x 4 + 6 x 3 + 3 x 2

f ( x ) = 4 x 5 − 12 x 4 + 9 x 3 f ( x ) = 4 x 5 − 12 x 4 + 9 x 3

f ( x ) = 2 x 4 ( x 3 − 4 x 2 + 4 x ) f ( x ) = 2 x 4 ( x 3 − 4 x 2 + 4 x )

f ( x ) = 4 x 4 ( 9 x 4 − 12 x 3 + 4 x 2 ) f ( x ) = 4 x 4 ( 9 x 4 − 12 x 3 + 4 x 2 )

For the following exercises, graph the polynomial functions. Note x - x - and y - y - intercepts, multiplicity, and end behavior.

f ( x ) = ( x + 3 ) 2 ( x − 2 ) f ( x ) = ( x + 3 ) 2 ( x − 2 )

g ( x ) = ( x + 4 ) ( x − 1 ) 2 g ( x ) = ( x + 4 ) ( x − 1 ) 2

h ( x ) = ( x − 1 ) 3 ( x + 3 ) 2 h ( x ) = ( x − 1 ) 3 ( x + 3 ) 2

k ( x ) = ( x − 3 ) 3 ( x − 2 ) 2 k ( x ) = ( x − 3 ) 3 ( x − 2 ) 2

m ( x ) = − 2 x ( x − 1 ) ( x + 3 ) m ( x ) = − 2 x ( x − 1 ) ( x + 3 )

n ( x ) = − 3 x ( x + 2 ) ( x − 4 ) n ( x ) = − 3 x ( x + 2 ) ( x − 4 )

For the following exercises, use the graphs to write the formula for a polynomial function of least degree.

For the following exercises, use the graph to identify zeros and multiplicity.

For the following exercises, use the given information about the polynomial graph to write the equation.

Degree 3. Zeros at x = –2, x = –2, x = 1, x = 1, and x = 3. x = 3. y -intercept at ( 0 , –4 ) . ( 0 , –4 ) .

Degree 3. Zeros at x = –5, x = –5, x = –2 , x = –2 , and x = 1. x = 1. y -intercept at ( 0 , 6 ) ( 0 , 6 )

Degree 5. Roots of multiplicity 2 at x = 3 x = 3 and x = 1 x = 1 , and a root of multiplicity 1 at x = –3. x = –3. y -intercept at ( 0 , 9 ) ( 0 , 9 )

Degree 4. Root of multiplicity 2 at x = 4, x = 4, and a roots of multiplicity 1 at x = 1 x = 1 and x = –2. x = –2. y -intercept at ( 0 , – 3 ) . ( 0 , – 3 ) .

Degree 5. Double zero at x = 1 , x = 1 , and triple zero at x = 3. x = 3. Passes through the point ( 2 , 15 ) . ( 2 , 15 ) .

Degree 3. Zeros at x = 4 , x = 4 , x = 3 , x = 3 , and x = 2. x = 2. y -intercept at ( 0 , −24 ) . ( 0 , −24 ) .

Degree 3. Zeros at x = −3 , x = −3 , x = −2 x = −2 and x = 1. x = 1. y -intercept at ( 0 , 12 ) . ( 0 , 12 ) .

Degree 5. Roots of multiplicity 2 at x = −3 x = −3 and x = 2 x = 2 and a root of multiplicity 1 at x = −2. x = −2.

y -intercept at ( 0 ,   4 ) . ( 0 ,   4 ) .

Degree 4. Roots of multiplicity 2 at x = 1 2 x = 1 2 and roots of multiplicity 1 at x = 6 x = 6 and x = −2. x = −2.

y -intercept at ( 0, 18 ) . ( 0, 18 ) .

Double zero at x = −3 x = −3 and triple zero at x = 0. x = 0. Passes through the point ( 1 , 32 ) . ( 1 , 32 ) .

For the following exercises, use a calculator to approximate local minima and maxima or the global minimum and maximum.

f ( x ) = x 3 − x − 1 f ( x ) = x 3 − x − 1

f ( x ) = 2 x 3 − 3 x − 1 f ( x ) = 2 x 3 − 3 x − 1

f ( x ) = x 4 + x f ( x ) = x 4 + x

f ( x ) = − x 4 + 3 x − 2 f ( x ) = − x 4 + 3 x − 2

f ( x ) = x 4 − x 3 + 1 f ( x ) = x 4 − x 3 + 1

For the following exercises, use the graphs to write a polynomial function of least degree.

Real-World Applications

For the following exercises, write the polynomial function that models the given situation.

A rectangle has a length of 10 units and a width of 8 units. Squares of x x by x x units are cut out of each corner, and then the sides are folded up to create an open box. Express the volume of the box as a polynomial function in terms of x . x .

Consider the same rectangle of the preceding problem. Squares of 2 x 2 x by 2 x 2 x units are cut out of each corner. Express the volume of the box as a polynomial in terms of x . x .

A square has sides of 12 units. Squares x + 1 x + 1 by x + 1 x + 1 units are cut out of each corner, and then the sides are folded up to create an open box. Express the volume of the box as a function in terms of x . x .

A cylinder has a radius of x + 2 x + 2 units and a height of 3 units greater. Express the volume of the cylinder as a polynomial function.

A right circular cone has a radius of 3 x + 6 3 x + 6 and a height 3 units less. Express the volume of the cone as a polynomial function. The volume of a cone is V = 1 3 π r 2 h V = 1 3 π r 2 h for radius r r and height h . h .

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Access for free at https://openstax.org/books/college-algebra-2e/pages/1-introduction-to-prerequisites

• Authors: Jay Abramson
• Publisher/website: OpenStax
• Book title: College Algebra 2e
• Publication date: Dec 21, 2021
• Location: Houston, Texas
• Book URL: https://openstax.org/books/college-algebra-2e/pages/1-introduction-to-prerequisites
• Section URL: https://openstax.org/books/college-algebra-2e/pages/5-3-graphs-of-polynomial-functions

© Jan 9, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

• Skip to main content

PAYMENT PLANS ARE NOW AVAILABLE • VISIT THE SHOP TO LEARN MORE!

All Things Algebra®

Algebra 2 Unit 5: Polynomial Functions

This unit includes 72 pages of guided notes, homework assignments, three quizzes, a study guide, and a unit test that cover the topics listed in the description below.

• Description
• Additional Information
• What Educators Are Saying

This unit contains the following topics:

• Operations with Monomials (exponent rules review) • Classifying Polynomials • Operations with Polynomials (add, subtract, multiply, divide by monomial) • Factoring Polynomials (includes GCF, difference of squares, sum of cubes, difference of cubes, trinomials, four terms) • Graphing Polynomial Functions • Identifying Key Characteristics of a Polynomial Function: domain, range, turning points (relative minimums and maximums), end behavior, increasing intervals, decreasing intervals zeros • Zeros of a Polynomial Function, Multiplicity, Effect of Multiplicity on a Graph • Solving Polynomials by Factoring • Dividing Polynomials (by factoring, long division, and synthetic division) • The Remainder Theorem • Operations with Functions • Compositions of Functions • Regression (review of linear/quadratic, cubic, quartic)

This unit does not contain activities.

This is the guided notes, homework assignments, quizzes, study guide, and unit test only.  For suggested activities to go with this unit, check out the ATA Activity Alignment Guides .

This resource is included in the following bundle(s):

Algebra 2 Curriculum

License Terms:

This purchase includes a single non-transferable license, meaning it is for one teacher only for personal use in their classroom and can not be passed from one teacher to another.  No part of this resource is to be shared with colleagues or used by an entire grade level, school, or district without purchasing the proper number of licenses.  A t ransferable license is not available for this resource.

Copyright Terms:

No part of this resource may be uploaded to the internet in any form, including classroom/personal websites or network drives, unless the site is password protected and can only be accessed by students.

What standards is this curriculum aligned to?

What format are the files in, will i have access to materials if they are updated, are answer keys included, are videos included.

LOVE these resources. They are so great when teaching, and interactive. The questions increasingly get more difficult at a great pace, and students are able to grasp the concepts and for those who need more examples there are plenty to chose from! Thank you!

-JENNIFER W.

Absolutely fantastic curriculum and explained in such an organized way for students to learn and use as a study tool. My students keep the reviews so they have reference material for college!

-CHRISTINE B.

Absolutely LOVED using this polynomials unit. The guided notes are so organized as are the answer keys. The provided quizzes and tests are editable which is great. I found my students struggling to finish the pre made assessments in our allotted time so the fact that it’s editable is amazing!

-JILLIAN C.

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

5.3: Graphs of Polynomial Functions

• Last updated
• Save as PDF
• Page ID 1675

Learning Objectives

• Recognize characteristics of graphs of polynomial functions.
• Use factoring to find zeros of polynomial functions.
• Identify zeros and their multiplicities.
• Determine end behavior.
• Understand the relationship between degree and turning points.
• Graph polynomial functions.
• Use the Intermediate Value Theorem.

The revenue in millions of dollars for a fictional cable company from 2006 through 2013 is shown in Table \(\PageIndex{1}\).

The revenue can be modeled by the polynomial function

\[R(t)=−0.037t^4+1.414t^3−19.777t^2+118.696t−205.332\]

where \(R\) represents the revenue in millions of dollars and \(t\) represents the year, with \(t=6\) corresponding to 2006. Over which intervals is the revenue for the company increasing? Over which intervals is the revenue for the company decreasing? These questions, along with many others, can be answered by examining the graph of the polynomial function. We have already explored the local behavior of quadratics, a special case of polynomials. In this section we will explore the local behavior of polynomials in general.

Recognizing Characteristics of Graphs of Polynomial Functions

Polynomial functions of degree 2 or more have graphs that do not have sharp corners; recall that these types of graphs are called smooth curves. Polynomial functions also display graphs that have no breaks. Curves with no breaks are called continuous. Figure \(\PageIndex{1}\) shows a graph that represents a polynomial function and a graph that represents a function that is not a polynomial.

Example \(\PageIndex{1}\): Recognizing Polynomial Functions

Which of the graphs in Figure \(\PageIndex{2}\) represents a polynomial function?

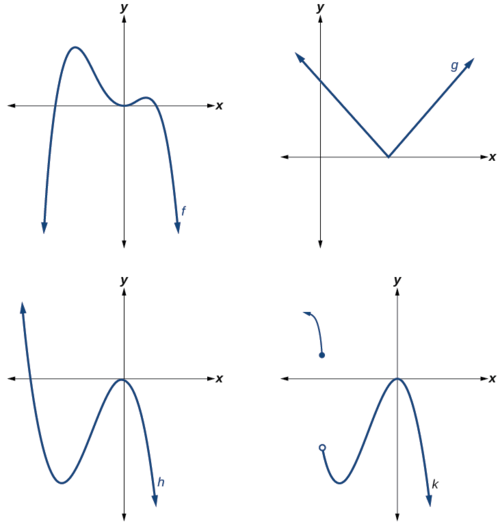
• The graphs of \(f\) and \(h\) are graphs of polynomial functions. They are smooth and continuous .
• The graphs of \(g\) and \(k\) are graphs of functions that are not polynomials. The graph of function \(g\) has a sharp corner. The graph of function \(k\) is not continuous.

Do all polynomial functions have as their domain all real numbers?

• Yes. Any real number is a valid input for a polynomial function.

Using Factoring to Find Zeros of Polynomial Functions

Recall that if \(f\) is a polynomial function, the values of \(x\) for which \(f(x)=0\) are called zeros of \(f\). If the equation of the polynomial function can be factored, we can set each factor equal to zero and solve for the zeros.

We can use this method to find x-intercepts because at the x-intercepts we find the input values when the output value is zero. For general polynomials, this can be a challenging prospect. While quadratics can be solved using the relatively simple quadratic formula, the corresponding formulas for cubic and fourth-degree polynomials are not simple enough to remember, and formulas do not exist for general higher-degree polynomials. Consequently, we will limit ourselves to three cases in this section:

The polynomial can be factored using known methods: greatest common factor and trinomial factoring. The polynomial is given in factored form. Technology is used to determine the intercepts.

HOwTO: Given a polynomial function \(f\), find the x-intercepts by factoring

• Set \(f(x)=0\).
• Factor out any common monomial factors.
• Factor any factorable binomials or trinomials.
• Set each factor equal to zero and solve to find the x-intercepts.

Example \(\PageIndex{2}\): Finding the x-Intercepts of a Polynomial Function by Factoring

Find the x-intercepts of \(f(x)=x^6−3x^4+2x^2\).

We can attempt to factor this polynomial to find solutions for \(f(x)=0\).

\[\begin{align*} x^6−3x^4+2x^2&=0 & &\text{Factor out the greatest common factor.} \\ x^2(x^4−3x^2+2)&=0 & &\text{Factor the trinomial.} \\ x^2(x^2−1)(x^2−2)&=0 & &\text{Set each factor equal to zero.} \end{align*}\]

\[\begin{align*} x^2&=0 & & & (x^2−1)&=0 & & & (x^2−2)&=0 \\ x^2&=0 & &\text{ or } & x^2&=1 & &\text{ or } & x^2&=2 \\ x&=0 &&& x&={\pm}1 &&& x&={\pm}\sqrt{2} \end{align*}\] .

This gives us five x-intercepts: \((0,0)\), \((1,0)\), \((−1,0)\), \((\sqrt{2},0)\),and \((−\sqrt{2},0)\) (Figure \(\PageIndex{3}\)). We can see that this is an even function.

Example \(\PageIndex{3}\): Finding the x-Intercepts of a Polynomial Function by Factoring

Find the x-intercepts of \(f(x)=x^3−5x^2−x+5\).

Find solutions for \(f(x)=0\) by factoring.

\[\begin{align*} x^3−5x^2−x+5&=0 &\text{Factor by grouping.} \\ x^2(x−5)−(x−5)&=0 &\text{Factor out the common factor.} \\ (x^2−1)(x−5)&=0 &\text{Factor the difference of squares.} \\ (x+1)(x−1)(x−5)&=0 &\text{Set each factor equal to zero.} \end{align*}\]

\[\begin{align*} x+1&=0 & &\text{or} & x−1&=0 & &\text{or} & x−5&=0 \\ x&=−1 &&& x&=1 &&& x&=5\end{align*}\]

There are three x-intercepts: \((−1,0)\), \((1,0)\), and \((5,0)\) (Figure \(\PageIndex{4}\)).

Example \(\PageIndex{4}\): Finding the y- and x-Intercepts of a Polynomial in Factored Form

Find the y- and x-intercepts of \(g(x)=(x−2)^2(2x+3)\).

The y-intercept can be found by evaluating \(g(0)\).

\[\begin{align*} g(0)&=(0−2)^2(2(0)+3) \\ &=12 \end{align*}\]

So the y-intercept is \((0,12)\).

The x-intercepts can be found by solving \(g(x)=0\).

\[(x−2)^2(2x+3)=0\]

\[\begin{align*} (x−2)^2&=0 & & & (2x+3)&=0 \\ x−2&=0 & &\text{or} & x&=−\dfrac{3}{2} \\ x&=2 \end{align*}\]

So the x-intercepts are \((2,0)\) and \(\left(−\dfrac{3}{2},0\right)\).

We can always check that our answers are reasonable by using a graphing calculator to graph the polynomial as shown in Figure \(\PageIndex{5}\).

Example \(\PageIndex{5}\): Finding the x-Intercepts of a Polynomial Function Using a Graph

Find the x-intercepts of \(h(x)=x^3+4x^2+x−6\).

This polynomial is not in factored form, has no common factors, and does not appear to be factorable using techniques previously discussed. Fortunately, we can use technology to find the intercepts. Keep in mind that some values make graphing difficult by hand. In these cases, we can take advantage of graphing utilities.

Looking at the graph of this function, as shown in Figure \(\PageIndex{6}\), it appears that there are x-intercepts at \(x=−3,−2, \text{ and }1\).

We can check whether these are correct by substituting these values for \(x\) and verifying that \[h(−3)=h(−2)=h(1)=0. \nonumber\]

Since \(h(x)=x^3+4x^2+x−6\), we have:

\[ \begin{align*} h(−3)&=(−3)^3+4(−3)^2+(−3)−6=−27+36−3−6=0 \\[4pt] h(−2) &=(−2)^3+4(−2)^2+(−2)−6 =−8+16−2−6=0 \\[4pt] h(1)&=(1)^3+4(1)^2+(1)−6=1+4+1−6=0 \end{align*}\]

Each x-intercept corresponds to a zero of the polynomial function and each zero yields a factor, so we can now write the polynomial in factored form.

\[\begin{align*} h(x)&=x^3+4x^2+x−6 \\ &=(x+3)(x+2)(x−1) \end{align*}\]

Exercise \(\PageIndex{1}\)

Find the y-and x-intercepts of the function \(f(x)=x^4−19x^2+30x\).

• y-intercept \((0,0)\);
• x-intercepts \((0,0)\), \((–5,0)\), \((2,0)\), and \((3,0)\)

Identifying Zeros and Their Multiplicities

Graphs behave differently at various x-intercepts. Sometimes, the graph will cross over the horizontal axis at an intercept. Other times, the graph will touch the horizontal axis and bounce off. Suppose, for example, we graph the function

\[f(x)=(x+3)(x−2)^2(x+1)^3.\]

Notice in Figure \(\PageIndex{7}\) that the behavior of the function at each of the x-intercepts is different.

The x-intercept −3 is the solution of equation \((x+3)=0\). The graph passes directly through thex-intercept at \(x=−3\). The factor is linear (has a degree of 1), so the behavior near the intercept is like that of a line—it passes directly through the intercept. We call this a single zero because the zero corresponds to a single factor of the function.

The x-intercept 2 is the repeated solution of equation \((x−2)^2=0\). The graph touches the axis at the intercept and changes direction. The factor is quadratic (degree 2), so the behavior near the intercept is like that of a quadratic—it bounces off of the horizontal axis at the intercept.

\[(x−2)^2=(x−2)(x−2)\]

The factor is repeated, that is, the factor \((x−2)\) appears twice. The number of times a given factor appears in the factored form of the equation of a polynomial is called the multiplicity . The zero associated with this factor, \(x=2\), has multiplicity 2 because the factor \((x−2)\) occurs twice.

The x-intercept −1 is the repeated solution of factor \((x+1)^3=0\).The graph passes through the axis at the intercept, but flattens out a bit first. This factor is cubic (degree 3), so the behavior near the intercept is like that of a cubic—with the same S-shape near the intercept as the toolkit function \(f(x)=x^3\). We call this a triple zero, or a zero with multiplicity 3.

For zeros with even multiplicities, the graphs touch or are tangent to the x-axis. For zeros with odd multiplicities, the graphs cross or intersect the x-axis. See Figure \(\PageIndex{8}\) for examples of graphs of polynomial functions with multiplicity 1, 2, and 3.

For higher even powers, such as 4, 6, and 8, the graph will still touch and bounce off of the horizontal axis but, for each increasing even power, the graph will appear flatter as it approaches and leaves the x-axis.

For higher odd powers, such as 5, 7, and 9, the graph will still cross through the horizontal axis, but for each increasing odd power, the graph will appear flatter as it approaches and leaves the x-axis.

Graphical Behavior of Polynomials at x-Intercepts

If a polynomial contains a factor of the form \((x−h)^p\), the behavior near the x-intercepth is determined by the power \(p\). We say that \(x=h\) is a zero of multiplicity \(p\).

The graph of a polynomial function will touch the x-axis at zeros with even multiplicities. The graph will cross the x-axis at zeros with odd multiplicities.

The sum of the multiplicities is the degree of the polynomial function.

HOWTO: Given a graph of a polynomial function of degree \(n\), identify the zeros and their multiplicities

• If the graph crosses the x-axis and appears almost linear at the intercept, it is a single zero.
• If the graph touches the x-axis and bounces off of the axis, it is a zero with even multiplicity.
• If the graph crosses the x-axis at a zero, it is a zero with odd multiplicity.
• The sum of the multiplicities is \(n\).

Example \(\PageIndex{6}\): Identifying Zeros and Their Multiplicities

Use the graph of the function of degree 6 in Figure \(\PageIndex{9}\) to identify the zeros of the function and their possible multiplicities.

The polynomial function is of degree \(n\). The sum of the multiplicities must be \(n\).

Starting from the left, the first zero occurs at \(x=−3\). The graph touches the x-axis, so the multiplicity of the zero must be even. The zero of −3 has multiplicity 2.

The next zero occurs at \(x=−1\). The graph looks almost linear at this point. This is a single zero of multiplicity 1.

The last zero occurs at \(x=4\).The graph crosses the x-axis, so the multiplicity of the zero must be odd. We know that the multiplicity is likely 3 and that the sum of the multiplicities is likely 6.

Exercise \(\PageIndex{2}\)

Use the graph of the function of degree 5 in Figure \(\PageIndex{10}\) to identify the zeros of the function and their multiplicities.

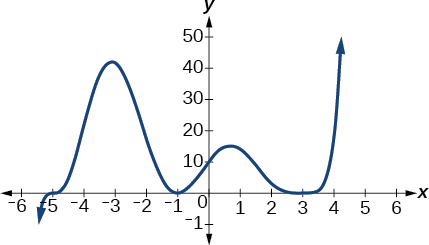
Figure \(\PageIndex{10}\): Graph of a polynomial function with degree 5.

The graph has a zero of –5 with multiplicity 1, a zero of –1 with multiplicity 2, and a zero of 3 with even multiplicity.

Determining End Behavior

As we have already learned, the behavior of a graph of a polynomial function of the form

\[f(x)=a_nx^n+a_{n−1}x^{n−1}+...+a_1x+a_0\]

will either ultimately rise or fall as \(x\) increases without bound and will either rise or fall as \(x\) decreases without bound. This is because for very large inputs, say 100 or 1,000, the leading term dominates the size of the output. The same is true for very small inputs, say –100 or –1,000.

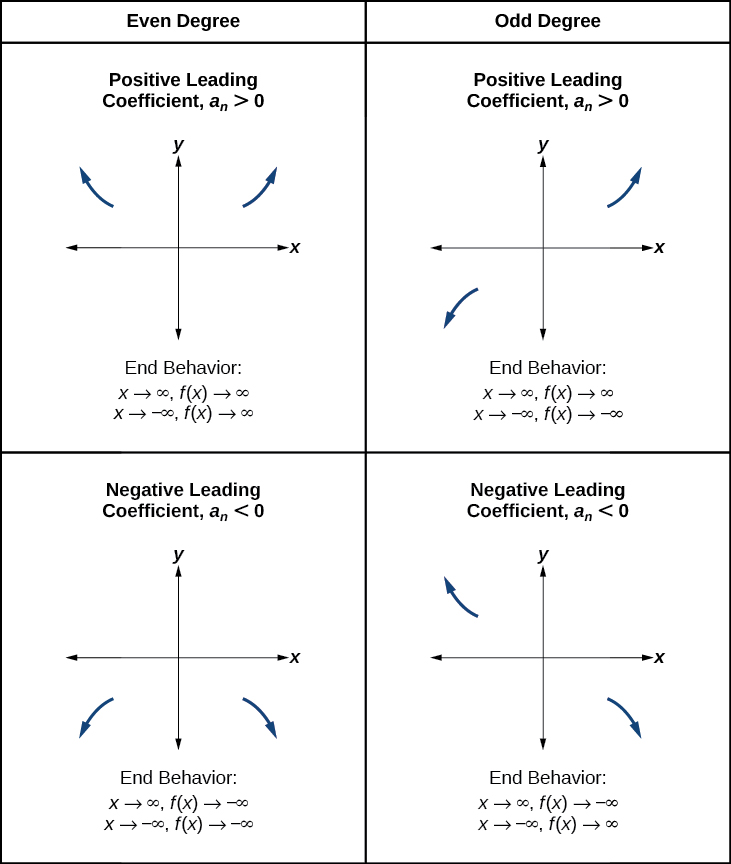
Recall that we call this behavior the end behavior of a function. As we pointed out when discussing quadratic equations, when the leading term of a polynomial function, \(a_nx^n\), is an even power function, as \(x\) increases or decreases without bound, \(f(x)\) increases without bound. When the leading term is an odd power function, as \(x\) decreases without bound, \(f(x)\) also decreases without bound; as \(x\) increases without bound, \(f(x)\) also increases without bound. If the leading term is negative, it will change the direction of the end behavior. Figure \(\PageIndex{11}\) summarizes all four cases.

Understanding the Relationship between Degree and Turning Points

In addition to the end behavior, recall that we can analyze a polynomial function’s local behavior. It may have a turning point where the graph changes from increasing to decreasing (rising to falling) or decreasing to increasing (falling to rising). Look at the graph of the polynomial function \(f(x)=x^4−x^3−4x^2+4x\) in Figure \(\PageIndex{12}\). The graph has three turning points.

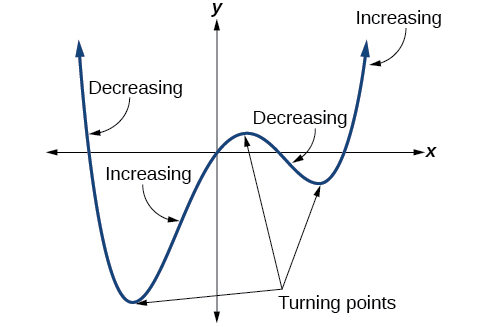
This function \(f\) is a 4th degree polynomial function and has 3 turning points. The maximum number of turning points of a polynomial function is always one less than the degree of the function.

Definition: Interpreting Turning Points

A turning point is a point of the graph where the graph changes from increasing to decreasing (rising to falling) or decreasing to increasing (falling to rising). A polynomial of degree \(n\) will have at most \(n−1\) turning points.

Example \(\PageIndex{7}\): Finding the Maximum Number of Turning Points Using the Degree of a Polynomial Function

Find the maximum number of turning points of each polynomial function.

• \(f(x)=−x^3+4x^5−3x^2+1\)
• \(f(x)=−(x−1)^2(1+2x^2)\)

a. \(f(x)=−x^3+4x^5−3x^2+1\)

First, rewrite the polynomial function in descending order: \(f(x)=4x^5−x^3−3x^2+1\)

Identify the degree of the polynomial function. This polynomial function is of degree 5.

The maximum number of turning points is \(5−1=4\).

b. \(f(x)=−(x−1)^2(1+2x^2)\)

First, identify the leading term of the polynomial function if the function were expanded.

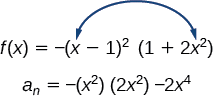
Then, identify the degree of the polynomial function. This polynomial function is of degree 4.

The maximum number of turning points is \(4−1=3\).

Graphing Polynomial Functions

We can use what we have learned about multiplicities, end behavior, and turning points to sketch graphs of polynomial functions. Let us put this all together and look at the steps required to graph polynomial functions.

Howto: Given a polynomial function, sketch the graph

• Find the intercepts.
• Check for symmetry. If the function is an even function, its graph is symmetrical about the y-axis, that is, \(f(−x)=f(x)\). If a function is an odd function, its graph is symmetrical about the origin, that is, \(f(−x)=−f(x)\).
• Use the multiplicities of the zeros to determine the behavior of the polynomial at the x-intercepts.
• Determine the end behavior by examining the leading term.
• Use the end behavior and the behavior at the intercepts to sketch a graph.
• Ensure that the number of turning points does not exceed one less than the degree of the polynomial.
• Optionally, use technology to check the graph.

Example \(\PageIndex{8}\): Sketching the Graph of a Polynomial Function

Sketch a graph of \(f(x)=−2(x+3)^2(x−5)\).

This graph has two x-intercepts. At \(x=−3\), the factor is squared, indicating a multiplicity of 2. The graph will bounce at this x-intercept. At \(x=5\),the function has a multiplicity of one, indicating the graph will cross through the axis at this intercept.

The y-intercept is found by evaluating \(f(0)\).

\[\begin{align*} f(0)&=−2(0+3)^2(0−5) \\ &=−2⋅9⋅(−5) \\ &=90 \end{align*}\]

The y-intercept is \((0,90)\).

Additionally, we can see the leading term, if this polynomial were multiplied out, would be \(−2x3\), so the end behavior is that of a vertically reflected cubic, with the outputs decreasing as the inputs approach infinity, and the outputs increasing as the inputs approach negative infinity. See Figure \(\PageIndex{13}\).

To sketch this, we consider that:

• As \(x{\rightarrow}−{\infty}\) the function \(f(x){\rightarrow}{\infty}\),so we know the graph starts in the second quadrant and is decreasing toward the x-axis.
• Since \(f(−x)=−2(−x+3)^2(−x–5)\) is not equal to \(f(x)\), the graph does not display symmetry.
• At \((−3,0)\), the graph bounces off of thex-axis, so the function must start increasing.
• At \((0,90)\), the graph crosses the y-axis at the y-intercept. See Figure \(\PageIndex{14}\).

Somewhere after this point, the graph must turn back down or start decreasing toward the horizontal axis because the graph passes through the next intercept at \((5,0)\). See Figure \(\PageIndex{15}\).

As \(x{\rightarrow}{\infty}\) the function \(f(x){\rightarrow}−{\infty}\),

so we know the graph continues to decrease, and we can stop drawing the graph in the fourth quadrant.

Using technology, we can create the graph for the polynomial function, shown in Figure \(\PageIndex{16}\), and verify that the resulting graph looks like our sketch in Figure \(\PageIndex{15}\).

Exercise \(\PageIndex{8}\)

Sketch a graph of \(f(x)=\dfrac{1}{4}x(x−1)^4(x+3)^3\).

Using the Intermediate Value Theorem

In some situations, we may know two points on a graph but not the zeros. If those two points are on opposite sides of the x-axis, we can confirm that there is a zero between them. Consider a polynomial function \(f\) whose graph is smooth and continuous. The Intermediate Value Theorem states that for two numbers \(a\) and \(b\) in the domain of \(f\),if \(a<b\) and \(f(a){\neq}f(b)\),then the function \(f\) takes on every value between \(f(a)\) and \(f(b)\). We can apply this theorem to a special case that is useful in graphing polynomial functions. If a point on the graph of a continuous function \(f\) at \(x=a\) lies above the x-axis and another point at \(x=b\) lies below thex-axis, there must exist a third point between \(x=a\) and \(x=b\) where the graph crosses the x-axis. Call this point \((c,f(c))\).This means that we are assured there is a solution \(c\) where \(f(c)=0\).

In other words, the Intermediate Value Theorem tells us that when a polynomial function changes from a negative value to a positive value, the function must cross the x-axis. Figure \(\PageIndex{18}\) shows that there is a zero between \(a\) and \(b\).

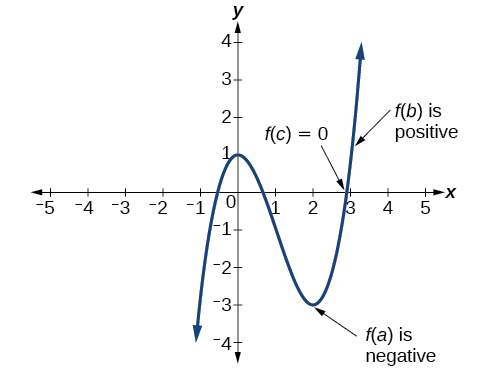
Definition: Intermediate Value Theorem

Let \(f\) be a polynomial function. The Intermediate Value Theorem states that if \(f(a)\) and \(f(b)\) have opposite signs, then there exists at least one value \(c\) between \(a\) and \(b\) for which \(f(c)=0\).

Example \(\PageIndex{9}\): Using the Intermediate Value Theorem

Show that the function \(f(x)=x^3−5x^2+3x+6\) has at least two real zeros between \(x=1\) and \(x=4\).

As a start, evaluate \(f(x)\) at the integer values \(x=1,\;2,\;3,\; \text{and }4\) (Table \(\PageIndex{2}\)).

We see that one zero occurs at \(x=2\). Also, since \(f(3)\) is negative and \(f(4)\) is positive, by the Intermediate Value Theorem, there must be at least one real zero between 3 and 4.

We have shown that there are at least two real zeros between \(x=1\) and \(x=4\).

We can also see on the graph of the function in Figure \(\PageIndex{19}\) that there are two real zeros between \(x=1\) and \(x=4\).

Exercise \(\PageIndex{4}\)

Show that the function \(f(x)=7x^5−9x^4−x^2\) has at least one real zero between \(x=1\) and \(x=2\).

Because \(f\) is a polynomial function and since \(f(1)\) is negative and \(f(2)\) is positive, there is at least one real zero between \(x=1\) and \(x=2\).

Writing Formulas for Polynomial Functions

Now that we know how to find zeros of polynomial functions, we can use them to write formulas based on graphs. Because a polynomial function written in factored form will have an x-intercept where each factor is equal to zero, we can form a function that will pass through a set of x-intercepts by introducing a corresponding set of factors.

Note: Factored Form of Polynomials

If a polynomial of lowest degree \(p\) has horizontal intercepts at \(x=x_1,x_2,…,x_n\), then the polynomial can be written in the factored form: \(f(x)=a(x−x_1)^{p_1}(x−x_2)^{p_2}⋯(x−x_n)^{p_n}\) where the powers \(p_i\) on each factor can be determined by the behavior of the graph at the corresponding intercept, and the stretch factor \(a\) can be determined given a value of the function other than the x-intercept.

• Identify the x-intercepts of the graph to find the factors of the polynomial.
• Examine the behavior of the graph at the x-intercepts to determine the multiplicity of each factor.
• Find the polynomial of least degree containing all the factors found in the previous step.
• Use any other point on the graph (the y-intercept may be easiest) to determine the stretch factor.

Example \(\PageIndex{10}\): Writing a Formula for a Polynomial Function from the Graph

Write a formula for the polynomial function shown in Figure \(\PageIndex{20}\).

This graph has three x-intercepts: \(x=−3,\;2,\text{ and }5\). The y-intercept is located at \((0,2)\).At \(x=−3\) and \( x=5\), the graph passes through the axis linearly, suggesting the corresponding factors of the polynomial will be linear. At \(x=2\), the graph bounces at the intercept, suggesting the corresponding factor of the polynomial will be second degree (quadratic). Together, this gives us

\[f(x)=a(x+3)(x−2)^2(x−5)\]

To determine the stretch factor, we utilize another point on the graph. We will use the y-intercept \((0,–2)\), to solve for \(a\).

\[\begin{align*} f(0)&=a(0+3)(0−2)^2(0−5) \\ −2&=a(0+3)(0−2)^2(0−5) \\ −2&=−60a \\ a&=\dfrac{1}{30} \end{align*}\]

The graphed polynomial appears to represent the function \(f(x)=\dfrac{1}{30}(x+3)(x−2)^2(x−5)\).

Exercise \(\PageIndex{5}\)

Given the graph shown in Figure \(\PageIndex{21}\), write a formula for the function shown.

\(f(x)=−\frac{1}{8}(x−2)^3(x+1)^2(x−4)\)

Using Local and Global Extrema

With quadratics, we were able to algebraically find the maximum or minimum value of the function by finding the vertex. For general polynomials, finding these turning points is not possible without more advanced techniques from calculus. Even then, finding where extrema occur can still be algebraically challenging. For now, we will estimate the locations of turning points using technology to generate a graph.

Each turning point represents a local minimum or maximum. Sometimes, a turning point is the highest or lowest point on the entire graph. In these cases, we say that the turning point is a global maximum or a global minimum . These are also referred to as the absolute maximum and absolute minimum values of the function.

Note: Local and Global Extrema

A local maximum or local minimum at \(x=a\) (sometimes called the relative maximum or minimum, respectively) is the output at the highest or lowest point on the graph in an open interval around \(x=a\).If a function has a local maximum at \(a\), then \(f(a){\geq}f(x)\)for all \(x\) in an open interval around \(x=a\). If a function has a local minimum at \(a\), then \(f(a){\leq}f(x)\)for all \(x\) in an open interval around \(x=a\).

A global maximum or global minimum is the output at the highest or lowest point of the function. If a function has a global maximum at \(a\), then \(f(a){\geq}f(x)\) for all \(x\). If a function has a global minimum at \(a\), then \(f(a){\leq}f(x)\) for all \(x\).

We can see the difference between local and global extrema in Figure \(\PageIndex{22}\).

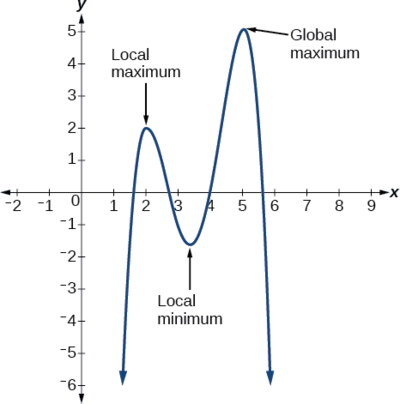
No. Only polynomial functions of even degree have a global minimum or maximum. For example, \(f(x)=x\) has neither a global maximum nor a global minimum.

Example \(\PageIndex{11}\): Using Local Extrema to Solve Applications

An open-top box is to be constructed by cutting out squares from each corner of a 14 cm by 20 cm sheet of plastic then folding up the sides. Find the size of squares that should be cut out to maximize the volume enclosed by the box.

We will start this problem by drawing a picture like that in Figure \(\PageIndex{23}\), labeling the width of the cut-out squares with a variable, \(w\).

Notice that after a square is cut out from each end, it leaves \(a(14−2w)\) cm by \((20−2w)\) cm rectangle for the base of the box, and the box will be \(w\) cm tall. This gives the volume

\[\begin{align*} V(w)&=(20−2w)(14−2w)w \\ &=280w−68w^2+4w^3 \end{align*}\]

Notice, since the factors are \(20–2w\), \(14–2w\), and \(w\), the three zeros are \(10, \,7,\) and \(0,) respectively. Because a height of \(0\) cm is not reasonable, we consider the only the zeros \(10\) and \(7.\) The shortest side is \(14\) and we are cutting off two squares, so values \(w\) may take on are greater than zero or less than \(7.\) This means we will restrict the domain of this function to \(0<w<7\).Using technology to sketch the graph of \(V(w)\) on this reasonable domain, we get a graph like that in Figure \(\PageIndex{24}\). We can use this graph to estimate the maximum value for the volume, restricted to values for \(w\) that are reasonable for this problem—values from 0 to 7.

From this graph, we turn our focus to only the portion on the reasonable domain, \([0, 7]\). We can estimate the maximum value to be around 340 cubic cm, which occurs when the squares are about 2.75 cm on each side. To improve this estimate, we could use advanced features of our technology, if available, or simply change our window to zoom in on our graph to produce Figure \(\PageIndex{25}\).

From this zoomed-in view, we can refine our estimate for the maximum volume to about 339 cubic cm, when the squares measure approximately 2.7 cm on each side.

Use technology to find the maximum and minimum values on the interval \([−1,4]\) of the function \(f(x)=−0.2(x−2)^3(x+1)^2(x−4)\).

The minimum occurs at approximately the point \((0,−6.5)\), and the maximum occurs at approximately the point \((3.5,7)\).

Key Concepts

• Polynomial functions of degree 2 or more are smooth, continuous functions.
• To find the zeros of a polynomial function, if it can be factored, factor the function and set each factor equal to zero.
• Another way to find the x-intercepts of a polynomial function is to graph the function and identify the points at which the graph crosses the x-axis.
• The multiplicity of a zero determines how the graph behaves at the x-intercepts.
• The graph of a polynomial will cross the horizontal axis at a zero with odd multiplicity.
• The graph of a polynomial will touch the horizontal axis at a zero with even multiplicity.
• The end behavior of a polynomial function depends on the leading term.
• The graph of a polynomial function changes direction at its turning points.
• A polynomial function of degree \(n\) has at most \(n−1\) turning points.
• To graph polynomial functions, find the zeros and their multiplicities, determine the end behavior, and ensure that the final graph has at most \(n−1\) turning points.
• Graphing a polynomial function helps to estimate local and global extremas.
• The Intermediate Value Theorem tells us that if \(f(a)\) and \(f(b)\) have opposite signs, then there exists at least one value \(c\) between \(a\) and \(b\) for which \(f(c)=0\).

global maximum highest turning point on a graph; \(f(a)\) where \(f(a){\geq}f(x)\) for all \(x\).

global minimum lowest turning point on a graph; \(f(a)\) where \(f(a){\leq}f(x)\) for all \(x\).

Intermediate Value Theorem for two numbers \(a\) and \(b\) in the domain of \(f\), if \(a<b\) and \(f(a){\neq}f(b)\), then the functionf takes on every value between \(f(a)\) and \(f(b)\); specifically, when a polynomial function changes from a negative value to a positive value, the function must cross the x-axis

multiplicity the number of times a given factor appears in the factored form of the equation of a polynomial; if a polynomial contains a factor of the form \((x−h)^p\), \(x=h\) is a zero of multiplicity \(p\).

• List of Lessons
• 1.1 Return to Algebra
• 1.2 Linear Inequalities
• 1.3 Absolute Value
• 1.4 Rewriting Equations
• Unit 1 Review
• Unit 1 Algebra Skillz Review
• 2.1 Function Notation
• 2.2 Functions/Relations
• 2.3 Slope and Rate of Change
• 2.4 Graphing Lines
• 2.5 Write Equations of Lines
• Unit 2 Review
• Unit 2 Algebra Skillz and SAT Review
• 3.1 Absolute Value Inequality
• 3.2 Absolute Value Graphs
• 3.3 Piecewise Functions
• Unit 3 Review
• Unit 3 Algebra Skillz
• 4.1 Solving Systems by Graphing
• 4.2 Solving Systems Algebraically
• 4.3 Systems of Inequalities
• Unit 4 Review
• 5.1 Graph in Vertex Form
• 5.2 Graph in Standard Form
• 5.3 Solve by Factoring
• 5.4 GCF and DoS
• 5.5 Solving by Square Roots
• Unit 5 Review
• Unit 5 Algebra Skillz and SAT Review
• 6.1 Imaginary and Complex Numbers
• 6.2 Operations on Complex Numbers
• 6.3 Completing the Square
• 6.4 Quadratic Formula
• Unit 6 Review
• Unit 6 Algebra Skillz
• SEMESTER EXAM
• 7.1 Properties of Exponents
• 7.2 Polynomial Division
• 7.3 Solving Polynomial Functions by Factoring
• 7.4 Graphs of Polynomial Functions
• 8.1 Evaluate Nth Roots
• 8.2 Properties of Rational Exponents
• 8.3 Function Operations and Composition
• 8.4 Inverse Operations
• 8.5 Graph Square and Cube Root Functions
• 8.6 Solving Radical Equations
• Unit 8 Review
• Unit 8 Algebra Skillz and SAT Review
• 9.1 Exponential Growth
• 9.2 Exponential Decay
• 9.3 The Number e
• 9.4 Intro to Logarithms
• 9.5 Properties of Logarithms
• 9.6 Solve Exponential and Log Equations
• Unit 9 Review
• Unit 9 Algebra Skillz
• 10.1 Graph Rational Functions
• 10.2 Multiply and Divide Rational Expressions
• 10.3 Add and Subtract Rational Expressions
• 10.4 Solve Rational Equations
• Unit 10 Review
• Unit 10 Algebra Skillz
• 11.1 Parabolas
• 11.2 Ellipses and Circles
• 11.3 Hyperbolas
• 11.4 Classifying Conics
• Unit 11 Review
• Unit 11 Algebra Skillz
• 12.1 Matrix Operations
• 12.2 Matrix Multiplication
• 12.3 Inverse Matrices
• 12.4 Encoding Messages
• Unit 12 Review
• SEMESTER 2 EXAM
• FlippedMath.com
• Teacher Resources

Section 7.2 Polynomial Functions

Practice solutions.

Corrective Assignment

Application Walkthrough

Leave any comments or questions below.  all comments will be approved before they are posted..

Have an account?

Unit 5 Polynomial Functions

10th - 11th grade, mathematics.

29 questions

Introducing new   Paper mode

No student devices needed.   Know more

• 1. Multiple Choice Edit 3 minutes 1 pt Factor n 2 + 5n - 6 (n - 2)(n - 3) (n - 1)(n + 6) (n + 1)(n - 6) (n + 2)(n - 3)
• 2. Multiple Choice Edit 3 minutes 1 pt Factor: x 2 - 9 (x + 3)(x - 3) (x + 3) 2 (x - 3) 2 (x+3)(x+3)
• 3. Multiple Choice Edit 3 minutes 1 pt x 2  + 81 Prime ( x - 9 ) ( x + 9 )  ( x + 9 ) ( x + 9 )  ( x - 9 ) ( x - 9 ) 
• 4. Multiple Choice Edit 3 minutes 1 pt (4x+1)(5x−2) 20x 2  + 3x − 2 20x 2  − 11x + 5 20x 2  − 18x + 2 20x 2  − 3x − 2
• 5. Multiple Choice Edit 3 minutes 1 pt (5x+1)(x+4) 5x 2   + 19x − 4 5x 2   + 21x + 4 5x 2  + 4 5x 2  − 19x − 4
• 6. Multiple Choice Edit 3 minutes 1 pt Fa  cto  r   x                2 -x-72 (x-9)(x+8) (x+9)(x-8) (x+9)(x-8) (x-9)(x-8)

Describe the end behavior of the graph.

x → -∞, f(x) → ∞ and x→∞, f(x) →⁻∞

x → -∞, f(x) → ∞ and x→∞, f(x) →∞

x → -∞, f(x) → ∞ and x→∞, f(x) → 0

x → -∞,f(x) → -∞and x→ ∞, f(x) → ∞

Write the polynomial function (in standard form) given the following zeros. x=-2, x=1, x= 4

f(x) = (x+2)(x-1)(x-4)

f(x) =x 3 - 3x 2 - 6x + 8

f(x) = x 3 + 8

f(x) = x 3 - 3x 2 + 8

• 19. Multiple Choice Edit 45 seconds 1 pt Find the sum. (2x 2 + 5x - 7) + ( 3 - 4x 2 + 6x) 2x 2  + 3x +1 -2x 2  - 11x -4 2x 2   + 5x -7 -2x 2 + 11x -4
• 20. Multiple Choice Edit 1 minute 1 pt Find the difference.  (3- 2x + 2x 2 ) - (4x -5 +3x 2 ) x 2 + 6x + 8 2x 2  + 5x - 7 -x 2   -6x + 8 -2x 2 + 11x - 4
• 21. Multiple Choice Edit 1 minute 1 pt Find the sum. (3y 2 + y 3 - 5) + (4y 2 -4y + 2y 3 + 8) 3y 3  + 7y 2  - 4y + 3 4y 2 + 3y 3 - y + 3 -y 4 + 2y 3  -y - 4 4y 3  + 3y 2  -3y + 1

Factor the following: x 2 +2x+xy+2y

(x 2 +2x)(xy+2y)

y(x 2 +2x+2)

Factor the following: n 2 +2n+3mn+6m

(n+2)(3m+n)

(n+2)+(3m+n)

2(3m+1)(n+m)

6m+(n 2 +2n+3)

Classify 4x 3 +5x 4 -6

Cubic trinomial

Quintic trinomial

Quartic trinomial

Quartic binomial

• 25. Multiple Choice Edit 5 minutes 1 pt (2x 3 - 5x - 7) ÷ (x - 2) 2x 3 - 4x 2 + 3x - 13 2x 3 + 4x 2 +3x - 1 2x 2 - 4x + 3 - 13/x-2 2x 2 + 4x + 3 - 1/x-2

4x 4 +4x 3 -9x 2 -x+2 ÷ (x-1)

4x 3 +8x 2 -7x-2

4x 3 +19x 2 -x-2

4x 3 +8x 2 -x-9

4x 3 +8x 2 -x-2

2x 3 + 5x 2 + 9 ÷ (x + 3)

2x 2 - x + 3

2x 3 - x 2 + 3x

2x 2 + 11x + 33 + 108/x+3

2x 2 - 5x + 12

• 28. Multiple Choice Edit 1 minute 1 pt What is another way to say "where a function crosses the x-axis"? x-intercept zero root all of these.

Write the polynomial in standard form given the following zeros. x = -2, 1, 4

Explore all questions with a free account

Continue with email

Continue with phone

Polynomial Functions Homework (Algebra 2 - Unit 5)

Also included in

Description

Polynomial Functions & Equations Homework Bundle:

This resource is a bundled set of homework practice sets and daily content quizzes for Unit 5: POLYNOMIAL FUNCTIONS & EQUATIONS designed for Algebra 2 Honors students. The file includes 27 pages of homework assignments and two different forms of a daily content quiz that you can use as a homework check, group work, or exit tickets.

The unit includes the following topics:

1) Polynomial Functions

2) Polynomials, Linear Factors, and Zeros

3) Solving Polynomial Equations

4) Dividing Polynomials

5) Theorems About Roots of Polynomials

6) The Fundamental Theorem of Algebra

7) The Binomial Theorem

8) Polynomials in the Real World

9) Transforming Polynomials

Click HERE to SAVE 20% by buying all POLYNOMIAL FUNCTIONS products, including cooperative activities, in UNIT 5 MEGA BUNDLE.

You may also be interested in:

Polynomial Sort and Match Activity

Polynomial Tournament Review Activity

Complex Roots Task Cards with QR Codes

SMART Board® ONLY

Foldables Only

Polynomial Essentials

Activities & Assessments Bundle

Here's a FREE item that complements the unit:

Remainder and Factor Theorems FREEBIE

****************************************************************

I LOVE FEEDBACK

Remember to leave feedback and you will earn points toward FREE TPT purchases. I love that feedback!

Also, follow me to be notified about my new products, sales, updates, and FREEBIES!

If you have any questions, comments or special requests

please contact me by email at: [email protected]

Thanks for shopping in my store!

Questions & Answers

Flamingo math by jean adams.

• We're hiring
• Help & FAQ
• Privacy policy
• Student privacy
• Terms of service
• Tell us what you think